In my opinion, you should try to solve the problem on your own at first and try to understand why there's a need for the disjoint set data structure. Essentially, the cases where matrix cell values are equal will become hard to assign a rank. There's a good explanation with visualization of it here - votrubac's answer: click me.

Essentially the case where disjoint-set is needed is shown below (credits to the author of this image - votrubac):

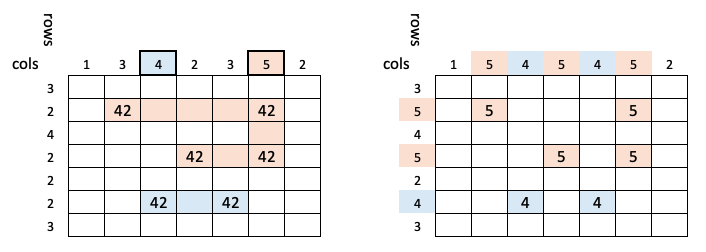
This helps a lot to understand the need for a disjoint-set.

DSA Pre-requisites

First of all, you need to know how a basic Disjoint-Set data structure (with Union-Find algorithm) works. You can learn it at GFG Union-Find

For shortening the runtime complexity, Union-Find is implemented with 2 optimizations: a. union by rank (which reduces union & find runtimes to O(log2(n)), and b. path compression (which reduces union & find time runtimes further to an amortized constant. You can learn it at GFG Union-Find by Rank

Logic

The logic is as below:

1. Store all the cells which have equal value together.
2. Traverse the cells from lowest to highest value (this is why a TreeMap is used to store)
3. Store max of each row and each col in an array (maxRowColValues) to compare and find the next max rank of a cell
4. For each group of cells with equal values (like for 42 in the image above):
   1. Create a disjoint set for each row and each col
   2. Unite x & y coordinates of each cell
   3. Form new groups of the united cells via their root cell (coordinatesByRoot)
   4. Assign rank + 1 to each group based on the max(rank) of the rows and cols they belong to
   5. Do not forget to increase the max of each row and each col (maxRowColValues)

Code (Java)

Note: A cell is referred to as coordinate below. I have tried to make the code as much understandable and maintainable as possible, yet all kinds of suggestions are welcome to make it even more understandable

public int[][] matrixRankTransform(int[][] matrix) {
    int rows = matrix.length, cols = matrix[0].length;
    int[][] rankMatrix = new int[rows][cols];

    // Create a TreeMap with the matrix cell values as keys since it stores keys in ASCENDING order
    TreeMap<Integer, ArrayList<Coordinate>> coordinatesByVal = new TreeMap<Integer, ArrayList<Coordinate>>();
    for (int r = 0; r < rows; r++) {
        for (int c = 0; c < cols; c++) {
            int val = matrix[r][c];
            if (coordinatesByVal.get(val) == null) {
                ArrayList<Coordinate> coordinates = new ArrayList<Coordinate>();
                coordinates.add(new Coordinate(r, c));
                coordinatesByVal.put(val, coordinates);
            } else {
                coordinatesByVal.get(val).add(new Coordinate(r, c));
            }
        }
    }

    // Create an array to store max for each row and col; cols start after rows
    int[] maxRowColValues = new int[rows + cols];

    // Assign ranks to coordinates of each val starting from lowest val
    for (int val: coordinatesByVal.keySet()) {
        ArrayList<Coordinate> coordinatesWithSameVal = coordinatesByVal.get(val);

        // Create a copy of maxRowColValues to make edits here; O(rows + cols)
        int[] maxRowColValuesClone = new int[rows + cols];
        for (int i = 0; i < maxRowColValues.length; i++) {
            maxRowColValuesClone[i] = maxRowColValues[i];
        }

        DisjointSet disjointSet = new DisjointSet(rows + cols); // each row and each col is now a subset of itself; O(rows + cols)

        for (Coordinate coordinate: coordinatesWithSameVal) {
            disjointSet.uniteSubsets(coordinate.x, coordinate.y + rows); // rows is added because 0 to rows-1 is for x-coordinates
            // Total time over complete matrix: O([rows + cols] log2([rows + cols]))
        }

        // Group coordinates with the same root
        HashMap<Node, ArrayList<Coordinate>> coordinatesByRoot = new HashMap<Node, ArrayList<Coordinate>>();
        for (Coordinate coordinate: coordinatesWithSameVal) {
            Node root = disjointSet.findRoot(coordinate.x); // y val is already united with x, so no need to find it
            if (coordinatesByRoot.get(root) == null) {
                ArrayList<Coordinate> nodes = new ArrayList<Coordinate>();
                nodes.add(coordinate);
                coordinatesByRoot.put(root, nodes);
            } else {
                coordinatesByRoot.get(root).add(coordinate);
            }
        }

        // Get maxRowColVal of each group and assign it to their rankMatrix cell
        for (Node root: coordinatesByRoot.keySet()) {
            ArrayList<Coordinate> groupCoordinates = coordinatesByRoot.get(root);

            // Get maxRowColVal of each group
            int maxRankOfGroup = 0;
            for (Coordinate coordinate: groupCoordinates) {
                int x = coordinate.x, y = coordinate.y;
                maxRankOfGroup = Math.max(maxRankOfGroup, Math.max(maxRowColValues[x], maxRowColValues[y + rows]));
            }

            // Assign new rank to each coordinate and then to all row-col values
            for (Coordinate coordinate: groupCoordinates) {
                System.out.println("Assigning rank " + (maxRankOfGroup + 1) + " to [" + coordinate.x + ", " + coordinate.y + "]");
                rankMatrix[coordinate.x][coordinate.y] = maxRankOfGroup + 1;
                maxRowColValues[coordinate.x] = maxRankOfGroup + 1;
                maxRowColValues[coordinate.y + rows] = maxRankOfGroup + 1;
            }
        }
    }
    return rankMatrix;
}

class Coordinate {
    int x, y;
    Coordinate(int x, int y) {
        this.x = x;
        this.y = y;
    }
}

class Node {
    int parent;
    int rank;

    Node(int parent, int rank) {
        this.parent = parent;
        this.rank = rank;
    }
}

class DisjointSet {
    Node[] subsets;

    DisjointSet(int numOfSubsetsAtStart) {
        this.subsets = new Node[numOfSubsetsAtStart];
        for (int i = 0; i < numOfSubsetsAtStart; i++) {
            subsets[i] = new Node(i, 1);
        }
    }

    // Find root parent of a number; this is essentially the "FIND" function of Union-Find
    Node findRoot(int num) {
        if (subsets[num].parent == num) {
            return subsets[num];
        } else {
            Node root = findRoot(subsets[num].parent);
            subsets[num].parent = root.parent; // This is path compression
            return root;
        }
    }

    // This is essentially "UNION BY RANK"; hence unions take log2(number of subsets)
    void uniteSubsets(int val1, int val2) {
        Node root1 = findRoot(val1);
        Node root2 = findRoot(val2);

        if (root1.rank < root2.rank) {
            // Since root2 has higher rank, root1 should be added as a child, otherwise root1's rank will be even higher
            root1.parent = root2.parent; // BTW root2.parent will always be its index in subsets array
            // No need to increase rank of root2, since its anyways higher
        } else if (root2.rank > root1.rank) {
            root2.parent = root1.parent; // No need to increase rank of root1, since its anyways higher
        } else {
            root1.parent = root2.parent;
            root2.rank++; // Both have same rank, hence the rank of the new parent needs to be increased
        }
    }
}

Happy coding!